|
(1) |
where  is the Riemann Zeta Function and
is the Riemann Zeta Function and  is the Gamma Function (Gradshteyn and Ryzhik
1980, p. 1076). The
is the Gamma Function (Gradshteyn and Ryzhik
1980, p. 1076). The  function satisfies the identity
function satisfies the identity
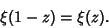 |
(2) |
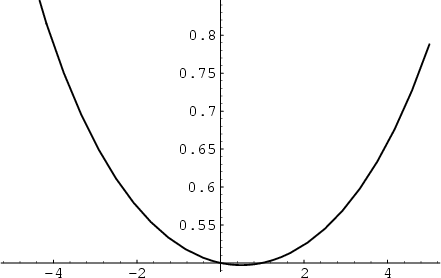
The zeros of  and of its Derivatives are all located on the Critical Strip
and of its Derivatives are all located on the Critical Strip  ,
where
,
where  . Therefore, the nontrivial zeros of the Riemann Zeta Function exactly correspond to those of
. Therefore, the nontrivial zeros of the Riemann Zeta Function exactly correspond to those of  .
The function
.
The function  is related to what Gradshteyn and Ryzhik (1980, p. 1074) call
is related to what Gradshteyn and Ryzhik (1980, p. 1074) call  by
by
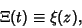 |
(3) |
where
 . This function can also be defined as
. This function can also be defined as
 |
(4) |
giving
 |
(5) |
The de Bruijn-Newman Constant is defined in terms of the  function.
function.
See also de Bruijn-Newman Constant
References
Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, corr. enl. 4th ed.
San Diego, CA: Academic Press, 1980.
© 1996-9 Eric W. Weisstein
1999-05-20