|
|
|

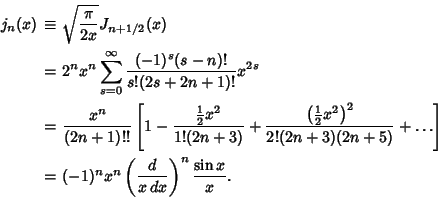
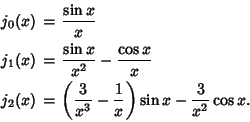
See also Poisson Integral Representation, Rayleigh's Formulas
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Spherical Bessel Functions.'' §10.1 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, pp. 437-442, 1972.
Arfken, G. ``Spherical Bessel Functions.'' §11.7 in
Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 622-636, 1985.